Calculus III Advanced (Course) (12.1) (Homework)

Section 12.1 Homework

From Calculus 10e by Larson and Edwards, p. 821. Exercises 2, 19, 20, 21, 22, 24, 52, 68, 72.

Exercise 12.1.2 Find the domain for the vector-valued function

\( \textbf{r}(t) = \sqrt{4-t^{2}}\textbf{i} + t^{2} \textbf{j} -6t \textbf{k} \)

Solution The parametric equations are:

\(f(t)=\sqrt{4-t^{2}} \), \(g(t)=t^{2}\), and \(h(t)=-6t\)

The functions \(g\) and \(h\) are continuous over the real numbers. The function \(f\) has the domain \([-2 \leqslant t \leqslant 2 ] \) because any value for \(t\) outside that range produces and irrational number. Therefore, the vector-valued function has the domain \([-2 \leqslant t \leqslant 2 ] \).

Exercise 12.1.19 Match the equation with its graph

\( \textbf{r}(t) = t\textbf{i} + 2t \textbf{j} + t^{2}\textbf{k} \)

Solution The three parametric equations are:

\(x=t\), \(y=2t\), and \(z=t^{2}\)

Figure 1 shows the graph which matches graph b.

Figure 1

Exercise 12.1.20 Match the equation with its graph

\( \textbf{r}(t) = \cos( \pi t)\textbf{i} + \sin( \pi t )\textbf{j} + t^{2}\textbf{k} \)

Solution The parametric equations are:

\( x= \cos( \pi t) \), \(y=\sin( \pi t ) \), and \(z=t^{2}\)

The match is graph c, as shown in Figure 2.

Figure 2

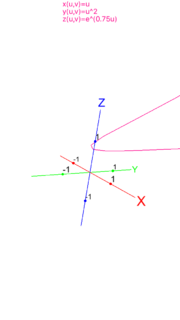
Exercise 12.1.21 Match the equation with its graph

\( \textbf{r}(t) = t\textbf{i} + t^{2}\textbf{j} + e^{0.75t}\textbf{k} \)

Solution The parametric equations are:

\( x= t\), \(y= t^{2} \), and \(z=e^{0.75t}\)

The match is graph d, as shown in Figure 4.

Figure 4

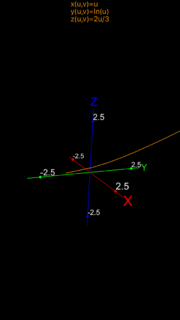
Exercise 12.1.22 Match the equation with its graph

\( \textbf{r}(t) = t\textbf{i} + \ln t \textbf{j} + \frac{2t}{3}\textbf{k} \)

Solution The parametric equations are:

\( x= t \), \(y=\ln t \), and \(z= \frac{2t}{3} \)

The match is graph a, as shown in Figure 3.

Figure 3

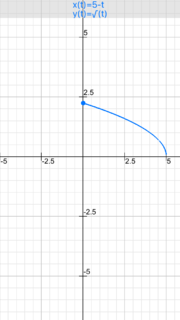
Exercise 12.1.24 Sketch the curve represented by the vector-valued function and give its orientation

\( \textbf{r}(t) = (5-t)\textbf{i} + \sqrt{ t }\textbf{j} \)

Solution The parametric equations are:

\(x=5-t\), and \(y=\sqrt{ t } \)

The curve traces from left to right, as shown in Table 1. The sketch is shown in Figure 5.

Table 1

t	(x,y)
0	\((5,\sqrt{5}) \)
1	\((4,\sqrt{4}) \)
2	\((3,\sqrt{3}) \)

Figure 5

Exercise 12.1.52 Representing a Graph by a Vector-Valued Function

$$ \frac{x^{2}}{9} + \frac{y^{2}}{16} = 1 $$

Solution Taking Example 12.1.1 and working backwards produces:

$$ \frac{x^{2}}{3^{2}}+ \frac{y^{2}}{4^{2}}=1.\:\:\:\: \color{red}{ \text{ The Cartesian equation}} $$

and

\( \textbf{r}(t)=3 \cos t \textbf{i} -4 \sin t\textbf{j},\: 0 \leqslant t \leqslant 2 \pi \:\:\:\:\)Vector-valued function

From Table 2 it is clear the trace starts at (3,0) and moves counter-clockwise, as shown in Figure 6.

Table 2

t	(x,y)
0	\((3, 0 ) \)
1	\((1.6, -3.3) \)

Figure 6

Exercise 12.1.68 Find the Limit

Find the limit, if it exists.

$$ \lim_{t \rightarrow \infty } \left( e^{-t}\textbf{i} + \frac{1}{t}\textbf{j} + \frac{t}{t^{2}+1}\textbf{k}\right)$$

Solution

$$ \lim_{t \rightarrow \infty } \left( e^{-t}\textbf{i} + \frac{1}{t}\textbf{j} + \frac{t}{t^{2}+1}\textbf{k}\right)$$

$$ =\lim_{t \rightarrow \infty } \left[ e^{-t}\right]\textbf{i} +\lim_{t \rightarrow \infty } \left[ \frac{1}{t}\right]\textbf{j} + \lim_{t \rightarrow \infty } \left[\frac{t}{t^{2}+1}\right]\textbf{k} $$

Distributive Property

Since the limit for component \( \textbf{j} \) does not exist when \( t=0\), the limit does not exist.

Exercise 12.1.72 Continuity for a Vector-Valued Function

Determine the interval(s) on which the vector-valued function is continuous.

$$\textbf{r}(t)= 2 e^{-t} \textbf{i} + e^{-t}\textbf{j} + \ln (t-1) \textbf{k} $$

Solution Component \( \textbf{k} \) is undefined when \(t=0\). Therefore the function is continuous when \(t \ne 0\).

Internal Links

Parent Article: Calculus III Advanced (Course)