Calculus III Advanced (Course) (12.5) (Homework)

Section 12.5 Homework

From Calculus 10e by Larson and Edwards, p. 860. Exercises 10, 12, 17.

Exercise 12.5.10 Finding the Arc Length for a Curve in three-dimensions

Sketch the curve and find its length over the given interval.

\( \textbf{r}(t) = \textbf{i} + t^{2}\textbf{j}+ t^{3}\textbf{k} \:\:\:\: [0,1]\)

Solution The sketch is shown in Figure 1. Finding the arc length. Applying differentiation to \(x(t)=1\), \(y(t)=t^{2}\), and \(z(t)=t^{3}\), produces -- \(x(t)=0\), \(y(t)=2t\), and \(z(t)=3t^{2}\).

\(s\)	$$= \int_{0}^{1} \sqrt{[x^{\prime}(t)]^{2} + [y^{\prime}(t)]^{2} + [z^{\prime}(t)]^{2} } \: dt \:\:\:\: \color{red}{ \text{Formula for arc length }} $$
	$$= \int_{0}^{1} \sqrt{ 0 + [2t]^{2} + [3t^{2}]^{2} } \: dt $$
	$$= \int_{0}^{1} \sqrt{ 4t^{2} + 9t^{4} } \: dt $$
	$$= \int_{0}^{1} \sqrt{ t^{2}(4 + 9t^{2}) } \: dt = 1.4397$$

Figure 1

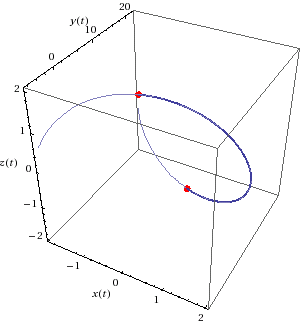
Exercise 12.5.12 Finding the Arc Length for a Curve in three-dimensions

Sketch the curve and find its length over the given interval.

\( \textbf{r}(t) = \langle 2 \sin t, 5t, 2 \cos t \rangle \:\:\:\: [0,\pi]\)

Solution The sketch is shown in Figure 1. Rewrite the equation as

\( \textbf{r}(t) = 2 \sin t \textbf{i} + 5t \textbf{j} + 2 \cos t \textbf{k} \).

Differentiation produces

\( \textbf{r}^{\prime}(t) = 2 \cos t \textbf{i} + 5 \textbf{j} - 2 \sin t \textbf{k} \),

where \(x^{\prime}(t)= 2 \cos t \), \(y^{\prime}(t)= 5\), \(z^{\prime}(t)= - 2 \sin t \).

\(s\)	$$= \int_{0}^{\pi} \sqrt{[x^{\prime}(t)]^{2} + [y^{\prime}(t)]^{2} + [z^{\prime}(t)]^{2} } \: dt \:\:\:\: \color{red}{ \text{Formula for arc length }} $$
	$$= \int_{0}^{\pi} \sqrt{ (2 \cos t)^{2} + 5^{2} - (2 \sin t)^{2} } \: dt $$
	$$= \int_{0}^{\pi} \sqrt{ 29 } \: dt = \sqrt{ 29 } \pi \approx 16.918$$

Figure 2

Exercise 12.5.17 Investigation

Consider the helix represented by the vector-valued function

\( \textbf{r}(t) = \langle 2 \cos t, 2 \sin t, t \rangle \)

(a)	Write the arc length \(s\) on the helix as a function for \(t\) by evaluating the integral $$s= \int_{0}^{t} \sqrt{[x^{\prime}(u)]^{2} + [y^{\prime}(u)]^{2} + [z^{\prime}(u)]^{2} } \: du. $$
(b)	Solve for \(t\) in the relationship derived in part (a), and substitute the result into the original parametric equations. This yields a parametrization for the curve in terms of the arc length parameter \(s\).
(c)	Find the coordinates for the point on the helix for arc lengths \(s=\sqrt{5}\) and \(s=4\).
(d)	Verify that \(\| \textbf{r}^{\prime}(t) \|=1\).

Solution Rewrite as

\( \textbf{r}(t) = 2 \cos t \textbf{i} + 2 \sin t \textbf{j} + t \textbf{k} \)

(a)		Differentiation produces
	\( \textbf{r}^{\prime}(t) \)	\( = -2 \sin t + 2 \cos t + 1 \)
	\( \| \textbf{r}^{\prime}(t) \| \)	\( = \sqrt{ (-2 \sin t)^{2} + (2 \cos t)^{2} + 1^{2} }\)
		\( = \sqrt{ 4 \sin^{2} t + 4 \cos^{2}t + 1 }\)
		\( = \sqrt{ 4( \sin^{2} t + \cos^{2}t ) + 1 }\)
		\( = \sqrt{ 4( 1 ) + 1 } = \sqrt{5} \)
		This produces
	\( s(t) \)	$$ \int_{0}^{t} \| \textbf{r}^{\prime}(u) \| \: du $$
		$$ \int_{0}^{t} \sqrt{5} \: du = \sqrt{5}t. $$
(b)		Plugging \( s= \sqrt{5}t \) or \( t= s/\sqrt{5} \) produces
	\( \textbf{r}(s) \)	$$= 2 \cos \left( \frac{1}{\sqrt{5}} s \right) \textbf{i} + 2 \sin \left( \frac{1}{\sqrt{5}}s \right) \textbf{j} + \frac{1}{\sqrt{5}}s \textbf{k} $$
(c)		Plugging \( s= \sqrt{5} \) and \( s= 4 \) produces
	\( \textbf{r}(\sqrt{5}) \)	$$= 2 \cos \left( \frac{1}{\sqrt{5}} \sqrt{5} \right) \textbf{i} + 2 \sin \left( \frac{1}{\sqrt{5}} \sqrt{5} \right) \textbf{j} + \frac{1}{\sqrt{5}}\sqrt{5} \textbf{k} $$
		\( = 2 \cos (1) + 2 \sin (1) + 1 \)
	\( \textbf{r}( 4 ) \)	$$= 2 \cos \left( \frac{4}{\sqrt{5}} \right) \textbf{i} + 2 \sin \left( \frac{4}{\sqrt{5}} \right) \textbf{j} + \frac{4}{\sqrt{5}} \textbf{k} $$
(d)
	\( \| \textbf{r}^{\prime}(s) \| \)	$$ = \sqrt{ \left(-\frac{2}{\sqrt{5}} \sin\left( \frac{1}{\sqrt{5}} s \right) \right)^{2} + \left(\frac{2}{\sqrt{5}} \cos \left( \frac{1}{\sqrt{5}} s \right) \right)^{2} + \left(\frac{1}{\sqrt{5}}\right)^{2} } $$
		$$ = \sqrt{ \frac{4}{5} \sin^{2}\left( \frac{\sqrt{5}}{5} s \right) + \frac{4}{5} \cos^{2} \left( \frac{\sqrt{5}}{5} s \right) + \frac{1}{5} } $$
		$$ = \sqrt{ \frac{4}{5} \left( \sin^{2}\left( \frac{\sqrt{5}}{5} s \right) + \cos^{2} \left( \frac{\sqrt{5}}{5} s \right) \right) + \frac{1}{5} } $$
		$$ = \sqrt{ \frac{4}{5}(1) + \frac{1}{5} } $$
		$$ = \sqrt{ \frac{4}{5} + \frac{1}{5} } = \sqrt{1} = 1 $$

Internal Links

Parent Article: Calculus III Advanced (Course)