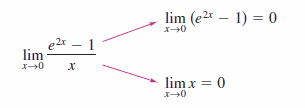Calculus II 08.07 Indeterminate Forms and L’Hôpital’s Rule

Previous	Calculus II 08.06 Integration by Tables and Other Integration Techniques
Next	Calculus II 08.08 Improper Integrals

8.7 Indeterminate Forms and L’Hôpital’s Rule[1]

• Recognize limits that produce indeterminate forms.
• Apply L’Hopital’s Rule to evaluate a limit.

Indeterminate Forms

The limit as \(x\) approaches 0 appears to be 2. Figure 8.7.1

Recall that the forms and \(0/0\) are \(\infty / \infty \) called indeterminate because they do not guarantee that a limit exists, nor do they indicate what the limit is, if one does exist. Solving indeterminate integrals required rewriting the expression by using various algebraic techniques. Table 8.7.1 Intermediate Forms Indeterminate Form Limit Algebraic Technique $$ \large \frac{0}{0} $$ $$ \lim_{x \to -1} \frac{2x^{2}-2}{x+1} $$ $$=\lim_{x \to -1} 2(x-1) $$ Divide the numerator and denominator by \( (x+1)\). $$ \large \frac{\infty}{\infty} $$ $$\lim_{x \to \infty } \frac{3x^{2}-1}{2x^{2}+1} $$ $$ =\lim_{x \to \infty} \frac{3-(1/x^{2})}{2+(1/x^{2})} =\frac{3}{2}$$ Divide the numerator and denominator by \( x^{2}\). These algebraic techniques can be extended to transcendental functions. Consider the limit $$ \large \lim_{x \to 0} \frac{e^{2x}-1}{e^{x}-1} $$ produces the indeterminate form \(0/0\). Factoring and then dividing produces $$ \large \lim_{x \to 0} \frac{e^{2x}-1}{e^{x}-1} $$ $$=\large \lim_{x \to 0} \frac{(e^{x}+1)(e^{x}-1)}{e^{x}-1} $$ $$ =\large \lim_{x \to 0} (e^{x}+1)=2. 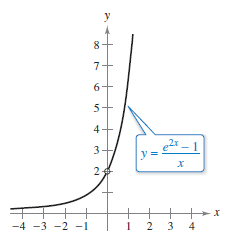$$ Not all indeterminate forms yield to algebraic techniques. Consider the case when a form has both algebraic and transcendental functions. Consider the limit $$ \large \lim_{x \to 0} \frac{e^{2x}-1}{x} $$ produces the indeterminate form \(0/0\). Rewriting the expression to obtain $$ \large \lim_{x \to 0} \left ( \frac{e^{2x}}{x} - \frac{1}{x} \right ) $$ merely produces another indeterminate form, \(\infty - \infty \). Using graphical techniques the limit appears to be 2, as shown in Figure 8.7.1 and the table below. This limit is verified in Example 8.7.1. Table 8.7.2 \(x\) -1 -0.1 -0.01 -0.001 0 0.001 0.01 0.1 1 $$ \frac{e^{2x}-1}{x} $$ 0.865 1.813 1.980 1.998  ? 2.002 2.020 2.214 6.389

L’Hôpital’s Rule

L’Hôpital’s Rule can find limits like those illustrated in Figure 8.7.1. This theorem states that under certain conditions, the limit for the quotient \(f(x)/g(x)\) is determined by the limit for the numerator's derivative over the denominator's derivative

$$ \frac{f'(x)}{g'(x)}. $$

Use the Extended Mean Value Theorem is used to prove L’Hôpital’s Rule.

Theorem 8.7.1 Extended Mean Value Theorem[2]

If \(f\) and \(g\) are differentiable on an open interval \((a,b)\) and continuous on \([a,b]\) such that \(g'(x) \neq 0 \) for any \(x\) in \((a,b)\), then there exists a point \(c\) in \((a,b)\) such that

$$ \large \frac{f'(c)}{g'(c)} = \frac{f(b)-f(a)}{g(b)-g(a)}. $$

To see why Theorem 8.7.1 is called the Extended Mean Value Theorem, consider the special case in which \(g(x)=x\). For this case, you obtain the standard Mean Value Theorem as presented in Section 3.2.

Theorem 8.7.2 L’Hôpital’s Rule

Let \(f\) and \(g\) be continuous and differentiable on an open interval \((a,b)\) containing \(c\), except possibly at \(c\) itself. Assume that \(g'(x) \neq 0\) for all \(x\) in \((a,b)\), except possibly at \(c\) itself. If the limit for \(f(x)/g(x)\) as \(x\) approaches \(c\) produces the indeterminate form \(0/0\) , then

$$ \large \lim_{x \to c} \frac{f(x)}{g(x)} = \frac{f'(x)}{g'(x)} $$

provided the limit on the right exists or is infinite. This result also applies when the limit for \(f(x)/g(x)\) as \(x\) approaches \(c\) produces thsee indeterminate forms \(\infty / \infty \), \( -\infty / \infty \), \(\infty / -\infty \), or \(-\infty / -\infty \).

Sometimes the Quotient Rule is incorrectly applied to \(f(x)/g(x)\). Be sure to remember

$$ \frac{f'(x)}{g'(x)} \neq \left ( \frac{f(x)}{g(x)} \right )'. $$

The rule can also be applied to one-sided limits. If the limit for \(f(x)/g(x)\) as \(x\) approaches \(c\) from the right produces the indeterminate form 0/0, then

$$ \large \lim_{x \to c^{+}} \frac{f(x)}{g(x)} = \lim_{x \to c^{+}} \frac{f'(x)}{g'(x)} $$

provided the limit exists or is infinite.

Example 8.7.1 Indeterminate Form 0/0

Evaluate

$$ \lim_{x \to 0} \frac{e^{2x}-1}{x}. $$

Solution Because direct substitution results in the indeterminate form 0/0 as shown in Figure 8.7.2, both limits exist, L’Hôpital’s Rule can be applied.

Figure 8.7.2

$$ \large \lim_{x \to 0} \frac{e^{2x}-1}{x} $$	$$ =\large \lim_{x \to 0} \frac{ \frac{d}{dx}[e^{2x}-1]}{\frac{d}{dx}[x]} \:\:\:\: $$	Apply L’Hôpital’s Rule
	$$ = \large \lim_{x \to 0} \frac{2e^{2x}}{1} \:\:\:\: $$	Differentiate numerator and denominator, Then evaluate the limit..
	$$ \large =2 $$	Evaluate the limit.

Example 8.7.2 Indeterminate Form \(\infty / \infty \)

Evaluate

$$ \lim_{x \to \infty } \frac{\ln x}{x}. $$

Solution Because direct substitution results in the indeterminate form \(\infty / \infty \) L’Hôpital’s Rule can be applied to produce

$$ \large \lim_{x \to \infty } \frac{\ln x}{x} $$	$$ = \large \lim_{x \to \infty } \frac{ \frac{d}{dx}[\ln x]}{\frac{d}{dx}[x]} \:\:\:\: $$	Apply L’Hôpital’s Rule
	$$ \large \lim_{x \to \infty } \frac{1}{x} \:\:\:\: $$	Differentiate numerator and denominator.
	$$= 0 $$	Evaluate the limit.

Example 8.7.3 Applying L’Hôpital’s Rule Recursively

Evaluate

$$ \lim_{x \to -\infty } \frac{x^{2}}{e^{-x}}. $$

Solution Because direct substitution results in the indeterminate form \(\infty / \infty \), L’Hôpital’s Rule can be applied.

$$ \large \lim_{x \to -\infty } \frac{x^{2}}{e^{-x}} = \lim_{x \to -\infty } \frac{ \frac{d}{dx}[x^{2}]}{\frac{d}{dx}[e^{-x}]} = \lim_{x \to -\infty } \frac{2x}{-e^{-x}}$$

This limit yields the indeterminate form \(-\infty / -\infty \) L’Hôpital’s Rule can be applied again

$$ \lim_{x \to -\infty } \frac{2x}{-e^{-x}} = \lim_{x \to -\infty } \frac{ \frac{d}{dx}[2x]}{\frac{d}{dx}[-e^{-x}]} = \lim_{x \to -\infty } \frac{2}{e^{-x}}=0.$$

Example 8.7.4 Indeterminate Form \(0 \cdot \infty \)

Evaluate

$$ \lim_{x \to \infty } e^{-x} \sqrt{-x}. $$

Because direct substitution produces the indeterminate form \(0 \cdot \infty \), rewrite the limit to fit the form 0/0 or \(\infty / \infty \). The limit can be rewritten to fit the second form.

$$ \lim_{x \to \infty } e^{-x} \sqrt{-x} = \large \lim_{x \to \infty } \frac {\sqrt{-x}}{e^{x}} $$

Now L’Hôpital’s Rule can be applied

$$ \large \lim_{x \to \infty } \frac {\sqrt{-x}}{e^{x}} $$	$$ \large = \lim_{x \to \infty } \frac{1/(2\sqrt{x}}{e^{x}}\:\:\:\: $$	Differentiate numerator and denominator.
	$$\large = \lim_{x \to \infty } \frac{1/(2\sqrt{x}}{e^{x}}$$	Simplify.
	$$=0.$$	Evaluate the limit.

The indeterminate forms \( 1^{\infty} \), \( \infty^{0}\), and \(0^{0}\) arise from limits that have variable bases and variable exponents. Previously logarithmic differentiation was used to find the derivative. A similar procedure is used when finding limits in Examples 8.7.5 through 7.

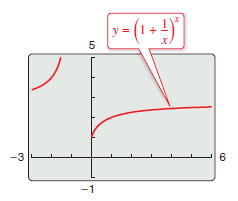
Example 8.7.5 Indeterminate Form \( 1^{\infty} \)

The limit for \([1+(1/x)]^{x}\) as \(x\) approaches infinity is \(e\). Figure 8.7.3

Evaluate $$ \lim_{x \to \infty } \left ( 1+ \frac{1}{x} \right )^{x}. $$ Solution Because direct substitution yields the indeterminate form \( 1^{\infty} \) assume that the limit exists and is equal to an unknown variable \(y\). $$ y = \lim_{x \to \infty } \left ( 1+ \frac{1}{x} \right )^{x} $$ Taking the natural logrithm for each side produces $$ \ln y = \ln \left[ \lim_{x \to \infty } \left ( 1+ \frac{1}{x} \right )^{x} \right ]. $$ Because the natural logarithmic function is continuous L’Hôpital’s Rule can be applied $$ \ln y $$ $$ = \ln \left [ \lim_{x \to \infty } \left ( 1+ \frac{1}{x} \right ) \right ] $$ Indeterminate form \( \infty \cdot 0 \) $$ = \lim_{x \to \infty } \left ( \frac{ \ln [1+(1/x)]}{1/x } \right ) \:\:\:\:$$ Indeterminate form \( 0 / 0 \) $$= \lim_{x \to \infty } \left ( \frac{ (-1/x^{2}) \left \{ 1/[1+(1/x)] \right \} }{-1/x^{2} } \right ) \:\:\:\: $$ L’Hôpital’s Rule $$ \large = \lim_{x \to \infty } \frac{ 1 }{1+ (1/x) } $$ $$= 1. $$ Thus \( \ln y = 1 \) and \(y=e\). Using back substitution produces $$ \large \lim_{x \to \infty } \left ( 1+ \frac{1}{x} \right )^{x} = e $$ The graphical solution is shown in Figure 8.7.3.

Example 8.7.6 Indeterminate Form \( 0^{0} \) from the Right Side

Evaluate $$ \large \lim_{x \to \infty^{+} } (\sin x )^{x}. $$ Solution Because direct substitution produces the indeterminate form \( 0^{0} \) assume that the limit exists and is equal to \(y\).

$$ \large y$$	$$ \large = \lim_{x \to \infty^{+} } (\sin x )^{x} $$	Indeterminate form \( 0^{0} \)
$$\large \ln y$$	$$ \large = \ln \left [ \lim_{x \to \infty^{+} } (\sin x )^{x} \right ] $$	Take natural log for each side
	$$ \large = \lim_{x \to \infty^{+} } \left [ \ln (\sin x )^{x} \right ] $$	Continuity
	$$ \large = \lim_{x \to \infty^{+} } \left [x \ln (\sin x ) \right ] \:\:\:\: $$	Indeterminate form \( 0 \cdot (- \infty ) \)
	$$ \large = \lim_{x \to \infty^{+} } \frac{ \ln (\sin x )}{1/x} $$	Indeterminate form \( - \infty /- \infty \)
	$$ \large = \lim_{x \to \infty^{+} } \frac{ \cot x }{-1/x^{2}} $$	L’Hôpital’s Rule
	$$ \large = \lim_{x \to \infty^{+} } \frac{ -x^{2} }{ \tan x} $$	Indeterminate form \( 0 / 0 \)
	$$ \large = \lim_{x \to \infty^{+} } \frac{ -2x }{ \sec^{2} x} $$	L’Hôpital’s Rule
	$$\large = 0$$

Thus \( \ln y = 0 \) and \(y=e^{0} = 1\). Using back substitution produces

$$ \large \lim_{x \to \infty^{+} } (\sin x )^{x} = 1. $$

Example 8.7.7 Indeterminate Form \( \infty - \infty \) from the Right Side

Evaluate $$ \large \lim_{x \to 1^{+} } \left ( \frac{1}{\ln x}- \frac{1}{x-1} \right ). $$ Solution Because direct substitution produces the indeterminate form \( \infty - \infty \) rewrite the form as two fractions so that L’Hôpital’s Rule can be applied.

$$ \large \lim_{x \to 1^{+} } \left ( \frac{1}{\ln x}- \frac{1}{x-1} \right ) = \lim_{x \to 1^{+} } \left [ \frac{x-1-\ln x}{(x-1) \ln x} \right ] $$

The new form has the indeterminate form \( 0 / 0 \) which yields to L’Hôpital’s Rule

$$ \large \lim_{x \to 1^{+} } \left ( \frac{1}{\ln x}- \frac{1}{x-1} \right )$$	$$ \large = \lim_{x \to 1^{+} } \frac{ \frac{d}{dx}[x-1-\ln x]}{\frac{d}{dx}[(x-1) \ln x]} $$
	$$ \large = \lim_{x \to 1^{+} } \left [ \frac{ 1- (1/x)}{(x-1)(1/x) + \ln x} \right ] $$
	$$ \large = \lim_{x \to 1^{+} } \left ( \frac{ x-1}{x-1 +x \ln x} \right ) $$

This limit also yields the indeterminate form \(0/0 \) so L’Hôpital’s Rule can be applied again to obtain

$$ \large \lim_{x \to 1^{+} } \left ( \frac{1}{\ln x}- \frac{1}{x-1} \right ) = \lim_{x \to 1^{+} } \left [ \frac{ 1}{1+x(1/x) + \ln x} \right ] = \frac{1}{2}$$

Internal Links

Parent Article: Calculus II 08 Integration Techniques