Calculus I 03.01 Extrema on an Interval

Previous	Calculus I 02 Differentiation
Next	Calculus I 03.02 Rolle’s Theorem and the Mean Value Theorem

3.1 Extrema on an Interval

• The definition for extrema function on an interval.
• The definition for relative extrema function on an open interval.
• Find extrema on a closed interval.

Figure 3.1.1

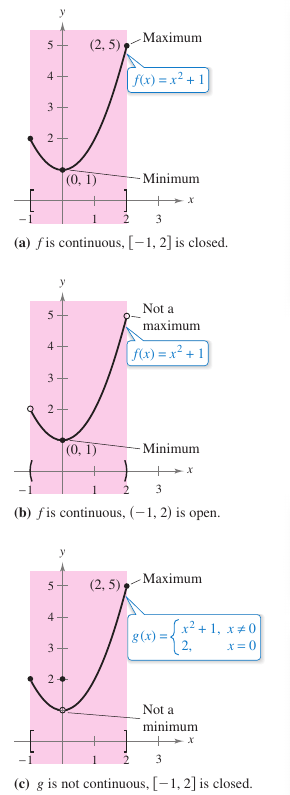
Extrema for a Function Calculus studies the how a function \(f\) behaves on an interval \(I\). Questions arise as to what is the maximum? Minimum? Where is the sharpest increase? The steepest decrease? This is called bounds checking or finding the extremes in a problem. This is called finding the extrema and has many applications in the real-world. Definition 3.1.1 Extrema Let \(f\) be defined on an interval \(I\) containing \(c\). 1. \(f(c)\) is the minimum \(f\) on \(I\) when \(f(c)\leq f(x)\) for all \(x\) in \(I\). 2. \(f(c)\) is the maximum \(f\) on \(I\) when \(f(c) \geq f(x)\) for all \(x\) in \(I\). The minimum and maximum for a function on an interval are the extreme values, or extrema, the singular form is extremum. They are also called the absolute minimum and absolute maximum, or the global minimum and global maximum. Extrema can occur at interior points or endpoints, as shown in Figure 3.1.1. Extrema that occur at the endpoints are called endpoint extrema. A function need not have a minimum or a maximum on an interval. For example, Figure 3.1.1(a) and (b), show the function \(f(x)=x^2+1\) has both a minimum and a maximum on the closed interval \([-1,2]\), but does not have a maximum on the open interval \((-1,2)\). Figure 3.1.1(c), shows the continuity, or the lack, can affect an extremums existence on the interval. This suggests Theorem 3.1.1 below. Theorem 3.1.1 The Extreme Value Theorem If \(f\) is continuous on a closed interval \([a,b]\), then \(f\) has both a minimum and maximum on the interval.

Relative Extrema and Critical Number

\(f\) has a relative maximum at \((0,0)\) and a relative minimum at \((2,-4)\). Figure 3.1.2 Figure 3.1.3

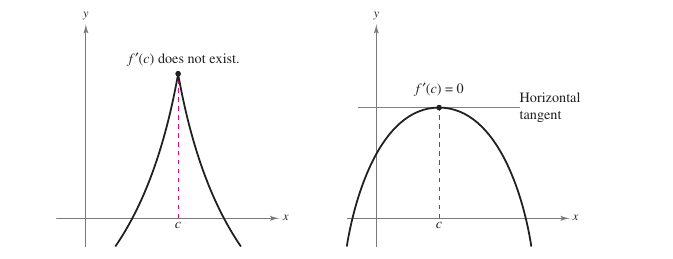
Informally, for a continuous function, the relative maximum occurs on a “hill” on the graph, and a relative minimum occurs in a “valley” on the graph. Such a hill and valley can occur in two ways. When the hill or valley is smooth and rounded, the graph has a horizontal tangent line at the high point or low point. When the hill or valley is sharp and peaked, the graph represents a function that is not differentiable at the high point or low point. Definition 3.1.2 Relative Extrema 1. If there is an open interval containing \(c\) on which \(f(c)\) is a maximum, then \(f(c)\) is called a relative maximum on \(f\), or you can say that \(f\) has a relative maximum at \((c,f(c))\). 2. If there is an open interval containing \(c\) on which \(f(c)\) is a minimum, then \(f(c)\) is called a relative minimum on \(f\), or you can say that \(f\) has a relative minimum at \((c,f(c))\). 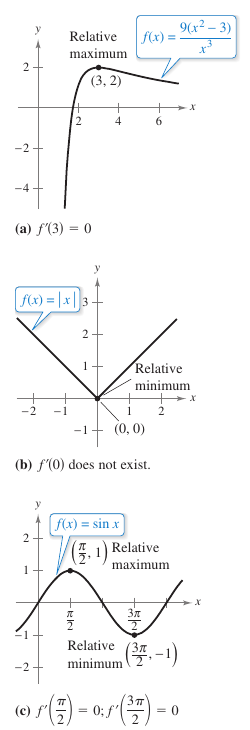The plural for relative maximum is relative maxima. The plural for relative minimum is relative minima. Relative maximum and relative minimum are sometimes called local maximum and local minimum, respectively. Example 3.1.1 examines the derivatives for functions at given relative extrema. Section 3.3 describes finding relative extrema. Example 3.1.1 Derivative Value at Relative Extrema Find the derivative value at each relative extremum shown in Figure 3.1.3. Solution a. The derivative is $$f(x)$$ $$=\frac{9(x^2-3)}{x^3}$$ \({f}'(x)\) $$=\frac{x^3(18x)-(9)(x^2-3)(3x^2)}{(x^3)^2}$$     Differentiate using the Quotient Rule. $$=\frac{9(9-x^2)}{x^4}.$$     Simplify. At the point \((3,2)\), the value for the derivative is \({f}'(3)=0\), as shown in Figure 3.1.3(a). b. At \(x=0\), the derivative for \(f(x)=\left |x \right |\) does not exist because the following one-sided limits differ, as shown in Figure 3.1.3(b). $$\lim_{x \to 0^-}\frac{f(x)-f(0)}{x-0}=\lim_{x \to 0^-}\frac{\left |x \right |}{x}=-1$$ Limit from the left $$\lim_{x \to 0^+}\frac{f(x)-f(0)}{x-0}=\lim_{x \to 0^+}\frac{\left |x \right |}{x}=1$$ Limit from the right c. The derivative for \(f(x)=\sin x\) is \({f}'(x)=\cos x.\) At the point \((\pi/2,1)\), the value for the derivative is \({f}'(\pi/2)=\cos (\pi/2)=0.\) At the point \((3\pi/2,-1)\), the value for the derivative is \({f}'(3\pi/2)=\cos (3\pi/2)=0\), as shown in Figure 3.1.3(c).

Note in Example 3.1.1 that at each relative extremum, the derivative either is zero or does not exist. The \(x\)-values at these special points are called critical numbers. Figure 3.1.4 illustrates both types. Notice in Definition 3.1.3 the critical number \(c\) must be in the domain for \(f\), but \(c\) does not have to be in the domain for \({f}'\).

Definition 3.1.3 Critical Number

Let \(f\) be defined at \(c\). If \({f}'(c)=0\) or if \(f\) is not differentiable at \(c\), then \(c\) is a critical number for \(f\).

\(c\) is a critical number for \(f\).
Figure 3.1.4

Theorem 3.1.2 Relative Extrema Occur Only at Critical Numbers

If \(f\) has a relative minimum or relative maximum at \(x=c\), then \(c\) is a critical number for \(f\).
Proof
Case 1: If \(f\) is not differentiable at \(x=c\), then by definition, \(c\) is a critical number for \(f\) and the theorem is valid.
Case 2: If \(f\) is differentiable at \(x=c\), then \({f}'(c)\) must be positive, negative, or 0.
Suppose \({f}'(c)\) is positive. Then

$${f}'(c)=\lim_{x \to c} \frac{f(x)-f(c)}{x-c} > 0$$

which implies there exists an interval \((a,b)\) containing \(c\) such that

$$\frac{f(x)-f(c)}{x-c} > 0, \text{ for all } x \neq c \text{ in } (a,b).$$

Because this quotient is positive, the signs for the denominator and numerator must agree. This produces the following inequalities for \(x\)-values in the interval \((a,b)\).

\(c\) from Left: \(x<c\) and \(f(x)<f(c) \rightarrow f(c)\) is not a relative minimum.

\(c\) from Right: \(x>c\) and \(f(x)>f(c) \rightarrow f(c)\) is not a relative maximum

The assumption that \({f}'(c)>0\) contradicts the hypothesis that \(f(c)\) is a relative extremum. Assuming that \({f}'(c)<0\) produces a similar contradiction, only one possibility is left, \({f}'(c)=0\). By definition \(c\) is a critical number for \(f\) and the theorem is valid.

Finding Extrema on a Closed Interval

Theorem 3.1.2 states that the relative extrema for a function can occur only at the critical numbers. The following guidelines describe how to find extrema on a closed interval.

Guidelines for Finding Extrema on a Closed Interval

To find the extrema for a continuous function \(f\) on a closed interval \([a,b]\) follow these steps. 1. Find the critical numbers for \(f\) in \((a,b)\).
2. Evaluate \(f\) at each critical number in \((a,b)\).
3. Evaluate \(f\) at each endpoint for \([a,b]\).
4. The least value is the minimum. The greatest is the maximum.
The next three examples apply these guidelines. Finding the critical numbers is only one part. Evaluating the function at the critical numbers and the endpoints is the other part.

Example 3.1.2 Finding Extrema on a Closed Interval

The closed interval \([-1,2]\), \(f\) has a minimum at \((1,-1)\) and a maximum at \((2,16)\). Figure 3.1.5

Find the extrema for \(f(x)=3x^4-4x^3\) on the interval \([-1,2]\). Solution Begin by differentiating the function \(f(x)\) \(=3x^4-4x^3\) \({f}'(x)\) \(=12x^3-12x^2\)     Differentiate To find the critical numbers for \(f\) in the interval \((-1,2)\) find all the \(x\)-values for which \({f}'(x)=0\) and for which \({f}'(x)\) does not exist. \(12x^3-12x^2\) \(=0\)     Set \({f}'(x)\) equal to 0. \(12x^2(x-1)\) \(=0\)     Factor \(\:\:x\) \(=0,1\)     Critical Numbers Because \({f}'\) is defined for all \(x\) conclude that these are the only critical numbers for \(f\). Evaluating \(f\) at the critical numbers and at the endpoints produces the results shown in Table 3.1.1 below. The graph for \(f\) is shown in Figure 3.1.5. Table 3.1.1 Left Endpoint Critical Number Critical Number Right Endpoint \(f(-1)=7\) \(f(0)=0\) \(f(1)=-1\) Minimum \(f(2)=16\) Maximum

In Figure 3.1.5, note that the critical number \(x=0\) does not yield a relative minimum or a relative maximum. This proves the converse for Theorem 3.1.2 is not true. In other words, the critical numbers for a function need not produce relative extrema.

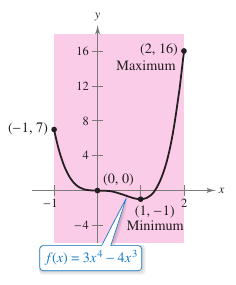
Example 3.1.3 Finding Extrema on a Closed Interval

On the closed interval \([-1,3]\), \(f\) has a minimum at \((-1,15)\) and a maximum at \((0,0)\). Figure 3.1.6

Find the extrema for \(f(x)=2x-3x^{2/3}\) on the interval \([-1,3]\). Solution Begin by differentiating the function \(f(x)\) \(=2x--3x^{2/3}\) \({f}'(x)\) $$=2-\frac{2}{x^{1/3}}$$     Differentiate $$=2 \left ( \frac{x^{1/3}-1}{x^{1/3}} \right )$$     Simplify. The derivative found two critical numbers on the interval \((-1,3)\). The number 1 is a critical number because \({f}'(1)=0\). The number 0 is a critical number because \({f}'(0)\) does not exist. Evaluating \(f\) at the critical numbers and endpoint produces the results shown in Table 3.1.2 below. The graph for \(f\) is shown in Figure 3.1.6. Table 3.1.2 Left Endpoint Critical Number Critical Number Right Endpoint \(f(-1)=-5\) Minimum \(f(0)=0\) Maximum \(f(1)=-1\) \(f(3)=6-3\sqrt[3]{9} \approx -0.24\)

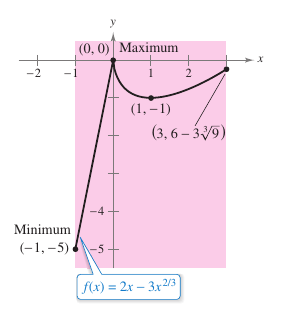
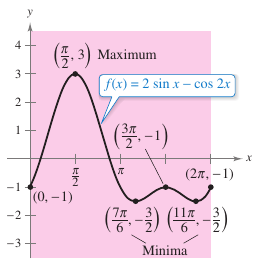
Example 3.1.4 Finding Extrema on a Closed Interval

On the closed interval \([0,2\pi]\), \(f\) has two minima at \((7\pi/6,-3/2)\) and \((11\pi/6,-3/2)\) and a maximum at \((\pi/2,3)\). Figure 3.1.7

Find the extrema for \(f(x)=2 \sin x - \cos 2x\) on the interval \([0,2\pi]\). Solution Begin by differentiating the function \(f(x)\) \(=2 \sin x - \cos 2x\) \({f}'(x)\) \(=2\cos x +2 \sin 2x\)     Differentiate \(=2 \cos x + 4 \cos x \sin x\)     \(\sin 2x=2 \cos x \sin x\) \(=2(\cos x)(1+2\sin x)\)     Factor. Because \(f\) is differentiable for all real \(x\) find all critical numbers for \(f\) by finding the zeros for derivative. Considering \(2(\cos x)(1+2\sin x)=0\) in the interval \((0,2\pi)\), the factor \(\cos x\) is zero when \(x=\pi/2\) and when \(x=3\pi/2\). The factor \((1+2 \sin x)\) is zero when \(x=7\pi/6\) and when \(x=11\pi/6\). Evaluating the four critical numbers and both endpoints is shown in Table 3.1.3 below. The graph for \(f\) is shown in Figure 3.1.7. Table 3.1.3 Left Endpoint Critical Number Critical Number Critical Number Critical Number Right Endpoint \(f(0)=-1\) $$f(\frac{\pi}{1}=3$$ Maximum $$f(\frac{7\pi}{6}=-\frac{3}{2}$$ Minimum $$f\frac{3\pi}{2}=-1$$ $$f\frac{11\pi}{6}=-\frac{3}{2}$$ \(f(2\pi)=-1\)

Internal Links

Parent Article: Calculus I 03 Differentiation Applications